add py3/node soln
This commit is contained in:
parent
56e8fc22e6
commit
1153573284
27
0669_trim-a-binary-search-tree/README.md
Normal file
27
0669_trim-a-binary-search-tree/README.md
Normal file
@ -0,0 +1,27 @@
|
|||||||
|
Given the `root` of a binary search tree and the lowest and highest boundaries as `low` and `high`, trim the tree so that all its elements lies in `[low, high]`. Trimming the tree should **not** change the relative structure of the elements that will remain in the tree (i.e., any node's descendant should remain a descendant). It can be proven that there is a **unique answer**.
|
||||||
|
|
||||||
|
Return _the root of the trimmed binary search tree_. Note that the root may change depending on the given bounds.
|
||||||
|
|
||||||
|
**Example 1:**
|
||||||
|
|
||||||
|
|
||||||
|
|
||||||
|
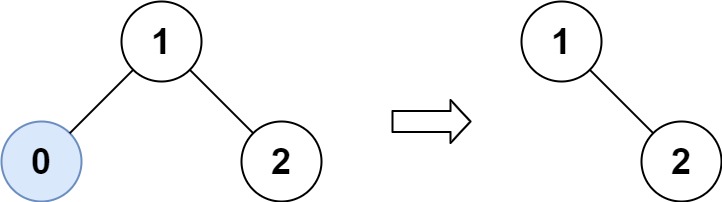
Input: root = [1,0,2], low = 1, high = 2
|
||||||
|
Output: [1,null,2]
|
||||||
|
|
||||||
|
|
||||||
|
**Example 2:**
|
||||||
|
|
||||||
|
|
||||||
|
|
||||||
|
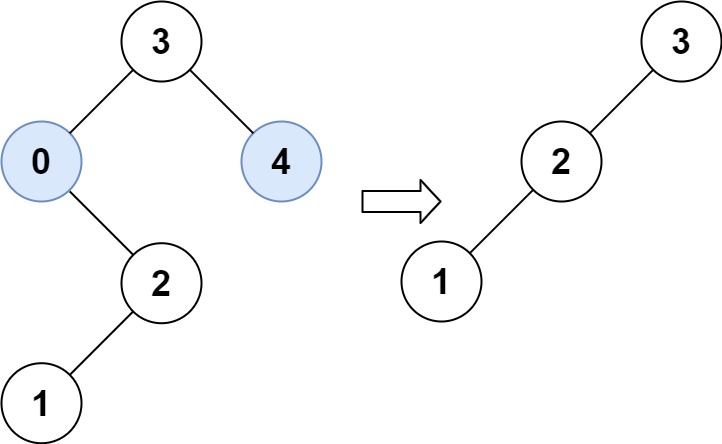
Input: root = [3,0,4,null,2,null,null,1], low = 1, high = 3
|
||||||
|
Output: [3,2,null,1]
|
||||||
|
|
||||||
|
|
||||||
|
**Constraints:**
|
||||||
|
|
||||||
|
* The number of nodes in the tree in the range `[1, 104]`.
|
||||||
|
* `0 <= Node.val <= 104`
|
||||||
|
* The value of each node in the tree is **unique**.
|
||||||
|
* `root` is guaranteed to be a valid binary search tree.
|
||||||
|
* `0 <= low <= high <= 104`
|
||||||
29
0669_trim-a-binary-search-tree/nodejs/solution.js
Normal file
29
0669_trim-a-binary-search-tree/nodejs/solution.js
Normal file
@ -0,0 +1,29 @@
|
|||||||
|
/**
|
||||||
|
* Definition for a binary tree node.
|
||||||
|
* function TreeNode(val, left, right) {
|
||||||
|
* this.val = (val===undefined ? 0 : val)
|
||||||
|
* this.left = (left===undefined ? null : left)
|
||||||
|
* this.right = (right===undefined ? null : right)
|
||||||
|
* }
|
||||||
|
*/
|
||||||
|
/**
|
||||||
|
* @param {TreeNode} root
|
||||||
|
* @param {number} low
|
||||||
|
* @param {number} high
|
||||||
|
* @return {TreeNode}
|
||||||
|
*/
|
||||||
|
function trimBST(root, low, high) {
|
||||||
|
if (!root) {
|
||||||
|
return null;
|
||||||
|
}
|
||||||
|
|
||||||
|
if (root.val < low) {
|
||||||
|
return trimBST(root.right, low, high);
|
||||||
|
} else if (root.val > high) {
|
||||||
|
return trimBST(root.left, low, high);
|
||||||
|
} else {
|
||||||
|
root.left = trimBST(root.left, low, high);
|
||||||
|
root.right = trimBST(root.right, low, high);
|
||||||
|
return root;
|
||||||
|
}
|
||||||
|
};
|
||||||
37
0669_trim-a-binary-search-tree/python3/solution.py
Normal file
37
0669_trim-a-binary-search-tree/python3/solution.py
Normal file
@ -0,0 +1,37 @@
|
|||||||
|
# Time: O(N)
|
||||||
|
# Space: O(N) due to call stack
|
||||||
|
|
||||||
|
# Definition for a binary tree node.
|
||||||
|
# class TreeNode:
|
||||||
|
# def __init__(self, val=0, left=None, right=None):
|
||||||
|
# self.val = val
|
||||||
|
# self.left = left
|
||||||
|
# self.right = right
|
||||||
|
class Solution:
|
||||||
|
def trimBST(self, root: Optional[TreeNode], low: int, high: int) -> Optional[TreeNode]:
|
||||||
|
# Recursion approach
|
||||||
|
|
||||||
|
def trim(node):
|
||||||
|
# Base case
|
||||||
|
if node is None:
|
||||||
|
return None
|
||||||
|
|
||||||
|
if node.val < low:
|
||||||
|
# Cause everything to the left will be
|
||||||
|
# less than `low` and hence trimmed
|
||||||
|
return trim(node.right)
|
||||||
|
|
||||||
|
elif node.val > high:
|
||||||
|
# Cause everything to the right will be
|
||||||
|
# greater than `high` and hence trimmed
|
||||||
|
return trim(node.left)
|
||||||
|
|
||||||
|
else:
|
||||||
|
# `node.val` is between [low, high] so
|
||||||
|
# just need to trim their left and right
|
||||||
|
# subtrees
|
||||||
|
node.left = trim(node.left)
|
||||||
|
node.right = trim(node.right)
|
||||||
|
return node
|
||||||
|
|
||||||
|
return trim(root)
|
||||||
Loading…
Reference in New Issue
Block a user